| comments | difficulty | edit_url | rating | source | tags | |||||
|---|---|---|---|---|---|---|---|---|---|---|
true |
困难 |
2020 |
第 123 场双周赛 Q4 |
|
给你一个 n x 2 的二维数组 points ,它表示二维平面上的一些点坐标,其中 points[i] = [xi, yi] 。
我们定义 x 轴的正方向为 右 (x 轴递增的方向),x 轴的负方向为 左 (x 轴递减的方向)。类似的,我们定义 y 轴的正方向为 上 (y 轴递增的方向),y 轴的负方向为 下 (y 轴递减的方向)。
你需要安排这 n 个人的站位,这 n 个人中包括 Alice 和 Bob 。你需要确保每个点处 恰好 有 一个 人。同时,Alice 想跟 Bob 单独玩耍,所以 Alice 会以 Alice 的坐标为 左上角 ,Bob 的坐标为 右下角 建立一个矩形的围栏(注意,围栏可能 不 包含任何区域,也就是说围栏可能是一条线段)。如果围栏的 内部 或者 边缘 上有任何其他人,Alice 都会难过。
请你在确保 Alice 不会 难过的前提下,返回 Alice 和 Bob 可以选择的 点对 数目。
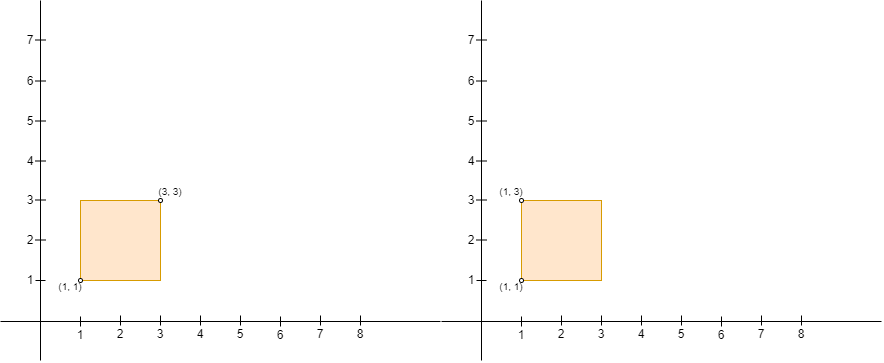
注意,Alice 建立的围栏必须确保 Alice 的位置是矩形的左上角,Bob 的位置是矩形的右下角。比方说,以 (1, 1) ,(1, 3) ,(3, 1) 和 (3, 3) 为矩形的四个角,给定下图的两个输入,Alice 都不能建立围栏,原因如下:
- 图一中,Alice 在
(3, 3)且 Bob 在(1, 1),Alice 的位置不是左上角且 Bob 的位置不是右下角。 - 图二中,Alice 在
(1, 3)且 Bob 在(1, 1),Bob 的位置不是在围栏的右下角。
示例 1:
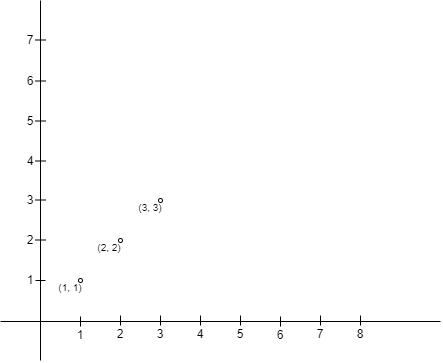
输入:points = [[1,1],[2,2],[3,3]] 输出:0 解释:没有办法可以让 Alice 的围栏以 Alice 的位置为左上角且 Bob 的位置为右下角。所以我们返回 0 。
示例 2:
输入:points = [[6,2],[4,4],[2,6]] 输出:2 解释:总共有 2 种方案安排 Alice 和 Bob 的位置,使得 Alice 不会难过: - Alice 站在 (4, 4) ,Bob 站在 (6, 2) 。 - Alice 站在 (2, 6) ,Bob 站在 (4, 4) 。 不能安排 Alice 站在 (2, 6) 且 Bob 站在 (6, 2) ,因为站在 (4, 4) 的人处于围栏内。
示例 3:
输入:points = [[3,1],[1,3],[1,1]] 输出:2 解释:总共有 2 种方案安排 Alice 和 Bob 的位置,使得 Alice 不会难过: - Alice 站在 (1, 1) ,Bob 站在 (3, 1) 。 - Alice 站在 (1, 3) ,Bob 站在 (1, 1) 。 不能安排 Alice 站在 (1, 3) 且 Bob 站在 (3, 1) ,因为站在 (1, 1) 的人处于围栏内。 注意围栏是可以不包含任何面积的,上图中第一和第二个围栏都是合法的。
提示:
2 <= n <= 1000points[i].length == 2-109 <= points[i][0], points[i][1] <= 109points[i]点对两两不同。
我们不妨考虑枚举矩形左上角的点
因此,我们对所有点按照
然后我们枚举左上角的点
枚举完所有的点对后,我们就得到了答案。
时间复杂度
class Solution:
def numberOfPairs(self, points: List[List[int]]) -> int:
points.sort(key=lambda x: (x[0], -x[1]))
ans = 0
for i, (_, y1) in enumerate(points):
max_y = -inf
for _, y2 in points[i + 1 :]:
if max_y < y2 <= y1:
max_y = y2
ans += 1
return ansclass Solution {
public int numberOfPairs(int[][] points) {
Arrays.sort(points, (a, b) -> a[0] == b[0] ? b[1] - a[1] : a[0] - b[0]);
int ans = 0;
int n = points.length;
final int inf = 1 << 30;
for (int i = 0; i < n; ++i) {
int y1 = points[i][1];
int maxY = -inf;
for (int j = i + 1; j < n; ++j) {
int y2 = points[j][1];
if (maxY < y2 && y2 <= y1) {
maxY = y2;
++ans;
}
}
}
return ans;
}
}class Solution {
public:
int numberOfPairs(vector<vector<int>>& points) {
sort(points.begin(), points.end(), [](const vector<int>& a, const vector<int>& b) {
return a[0] < b[0] || (a[0] == b[0] && b[1] < a[1]);
});
int n = points.size();
int ans = 0;
for (int i = 0; i < n; ++i) {
int y1 = points[i][1];
int maxY = INT_MIN;
for (int j = i + 1; j < n; ++j) {
int y2 = points[j][1];
if (maxY < y2 && y2 <= y1) {
maxY = y2;
++ans;
}
}
}
return ans;
}
};func numberOfPairs(points [][]int) (ans int) {
sort.Slice(points, func(i, j int) bool {
return points[i][0] < points[j][0] || points[i][0] == points[j][0] && points[j][1] < points[i][1]
})
for i, p1 := range points {
y1 := p1[1]
maxY := math.MinInt32
for _, p2 := range points[i+1:] {
y2 := p2[1]
if maxY < y2 && y2 <= y1 {
maxY = y2
ans++
}
}
}
return
}function numberOfPairs(points: number[][]): number {
points.sort((a, b) => (a[0] === b[0] ? b[1] - a[1] : a[0] - b[0]));
const n = points.length;
let ans = 0;
for (let i = 0; i < n; ++i) {
const [_, y1] = points[i];
let maxY = -Infinity;
for (let j = i + 1; j < n; ++j) {
const [_, y2] = points[j];
if (maxY < y2 && y2 <= y1) {
maxY = y2;
++ans;
}
}
}
return ans;
}public class Solution {
public int NumberOfPairs(int[][] points) {
Array.Sort(points, (a, b) => a[0] == b[0] ? b[1] - a[1] : a[0] - b[0]);
int ans = 0;
int n = points.Length;
int inf = 1 << 30;
for (int i = 0; i < n; ++i) {
int y1 = points[i][1];
int maxY = -inf;
for (int j = i + 1; j < n; ++j) {
int y2 = points[j][1];
if (maxY < y2 && y2 <= y1) {
maxY = y2;
++ans;
}
}
}
return ans;
}
}