常微分方程简介

docs/blog/posts/基于优化的轨迹规划.md

@@ -0,0 +1,39 @@
+---
+title: 基于优化的轨迹规划
+date:
+  created: 2024-10-30
+  updated: 2024-10-30
+categories:
+  - 机器人
+  - 移动机器人运动规划
+---
+
+## 1. 全局方法 和 局部方法
+
+![](https://picgo-1257309505.cos.ap-guangzhou.myqcloud.com/20241030211947.png)
+
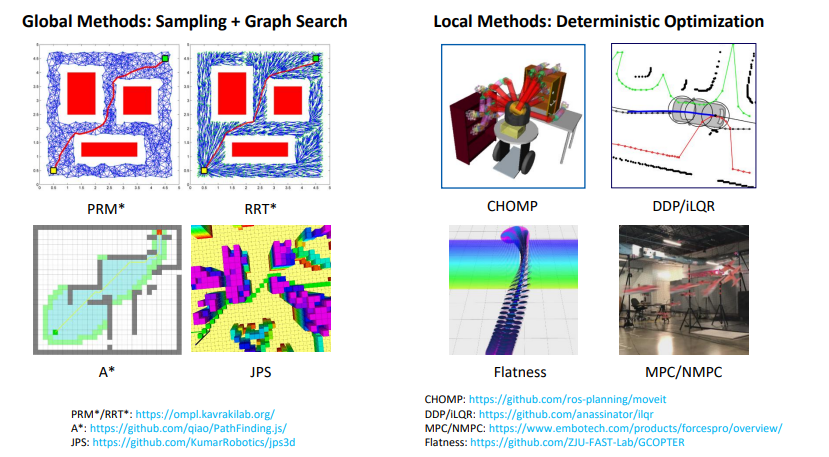+<!-- more -->
+
+## 2. 轨迹规划
+
+### 2.1 什么是轨迹
+
+时间参数化的路径
+
+### 2.2 平滑意味着什么
+
+1. 满足动力学约束 $\dot{x} = f(x, u)$
+2. 最小化能量泛函 $min \int_{t_0}^{t_f} L(x(t), u(t)) dt$
+
+### 2.3 为什么需要轨迹优化
+
+1. 能量最优
+2. 时间最优
+3. 驱动器限制
+4. 任务需求 (保持fov)
+
+## 3. 微分平坦(Differential Flatness)
+
+![](https://picgo-1257309505.cos.ap-guangzhou.myqcloud.com/20241106115907.png)
+
+微分平坦是使用系统的输出和输出的导数对系统的状态$x$和输入$u$进行描述，从而把系统方程$\dot{x} = f(x) + g(x)u$约束消掉

docs/blog/posts/常微分方程简介.md

@@ -0,0 +1,48 @@
+---
+title: 常微分方程简介
+date:
+  created: 2024-11-22
+  updated: 2024-11-26
+categories:
+  - 数学
+---
+
+## 1. 常微分方程的定义
+
+k阶常微分方程 (ODE of order k):
+
+$$ F(t, x, \dot{x}, ..., x^{(k)}) = 0 $$
+
+### 1.1 autonomous ODE
+
+- **autonomous** ODE:  $\dot{x} = w(t, x)$
+- non-autonomous ODE:  $\dot{x} = v(x)$
+
+### 1.2 homogeneous ODE
+
+- **homogeneous** ODE:  $\dot{x} = A(t)v(x)$
+- non-homogeneous ODE:  $\dot{x} = A(t)v(x) + B(t)$
+
+### 1.3 linear ODE
+
+- **linear** ODE:  $\dot{x} = A(t)x + B(t)$
+
+<!-- more -->
+
+!!! note "Lipschitz Continuity"
+
+    $$ |f(z) - f(y)| \leq L|z - y| $$
+
+!!! note "Banach Fixed Point Theorem"
+
+    ![](https://picgo-1257309505.cos.ap-guangzhou.myqcloud.com/20241122181656.png)
+
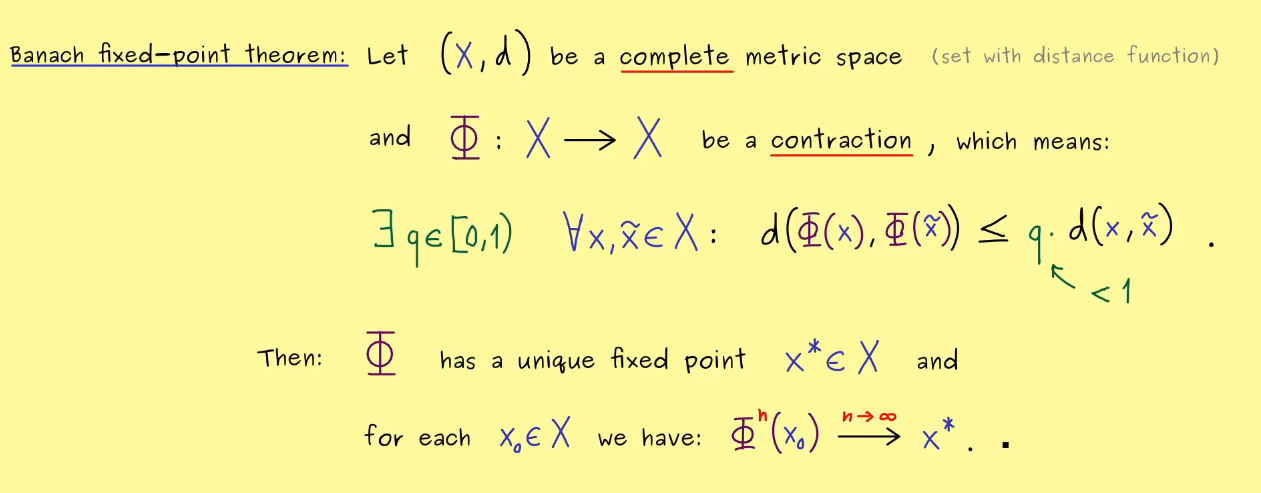+!!! note "Picard-Lindelöf Theorem"
+
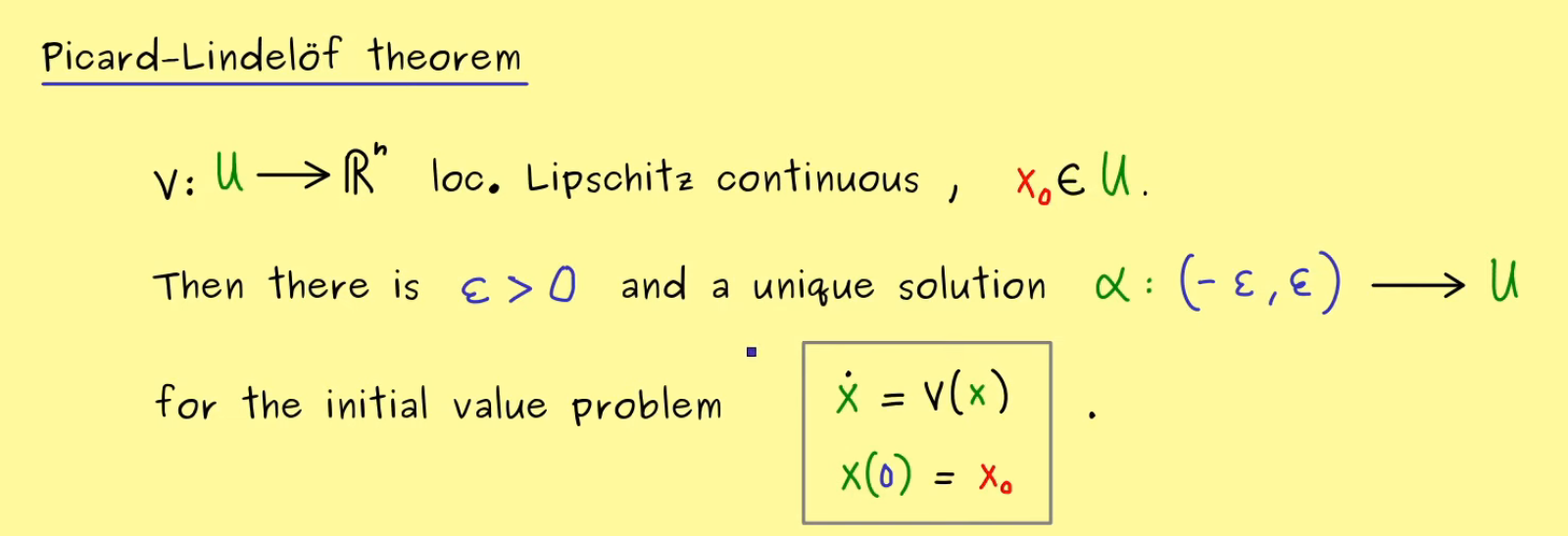+    ![](https://picgo-1257309505.cos.ap-guangzhou.myqcloud.com/20241122200147.png)
+
+![](https://picgo-1257309505.cos.ap-guangzhou.myqcloud.com/20241125121905.png)
+
+![](https://picgo-1257309505.cos.ap-guangzhou.myqcloud.com/20241125122011.png)
+
+![](https://picgo-1257309505.cos.ap-guangzhou.myqcloud.com/20241125210032.png)